Welcome to MASE Economics! In this blog post, we look at the main macroeconomic curves. Like the Phillips and Laffer curves, this curve is more than just a line on a graph. It is a powerful tool that opens a deeper understanding of important economic relationships such as inflation, unemployment, taxation, and trade.
Are you ready to jump into the first curve? Because we start with the Phillips curve!
phillips curve
The Phillips curve, named after New Zealand economist William Phillips, appeared in the late 1950s. From 1861 to 1957, Phillips observed a stable inverse relationship between unemployment and wage inflation in Britain. This groundbreaking discovery laid the foundation for our modern understanding of the Phillips curve.
The Phillips curve shows the trade-off between unemployment and inflation in the short run. Low unemployment rates are usually associated with high inflation rates and vice versa. The intuition behind this relationship is that as unemployment declines, workers gain bargaining power, leading to wage increases and thus prices.
The Phillips curve above shows a downward curve representing the inverse relationship between the inflation rate (vertical axis) and the unemployment rate (horizontal axis). The curves (A and B) highlight two points. A represents high inflation and low unemployment, and B represents low inflation and high unemployment. This visual representation reinforces the idea of a balance between two economic factors. The trade-off may not be sustainable in the long run. Economists say long-term inflation expectations are effect The position of the curve.
Although the Phillips curve was widely accepted in the 1960s, it faced challenges in the following decades. The stagflation of the 1970s, characterized by high unemployment and high inflation, contradicted the traditional Phillips curve relationship. This led to a reassessment of the stability of the curve and recognition of other factors that can influence unemployment-inflation dynamics, such as inflation expectations and supply shocks.
wrapper curve
The Laffer Curve, named after American economist Arthur Laffer, became famous in the late 1970s. Laffer’s concept emphasized the relationship between tax rates and government revenues, challenging the conventional wisdom that higher tax rates always lead to higher revenues.
The Laffer Curve shows that tax revenue initially increases as the tax rate increases from zero. However, beyond a certain point (the peak of the curve), high tax rates can reduce economic activity by discouraging people from working, investing and producing, which in turn can lead to lower tax revenues. This curve indicates that there is an optimal tax rate that maximizes government revenue.
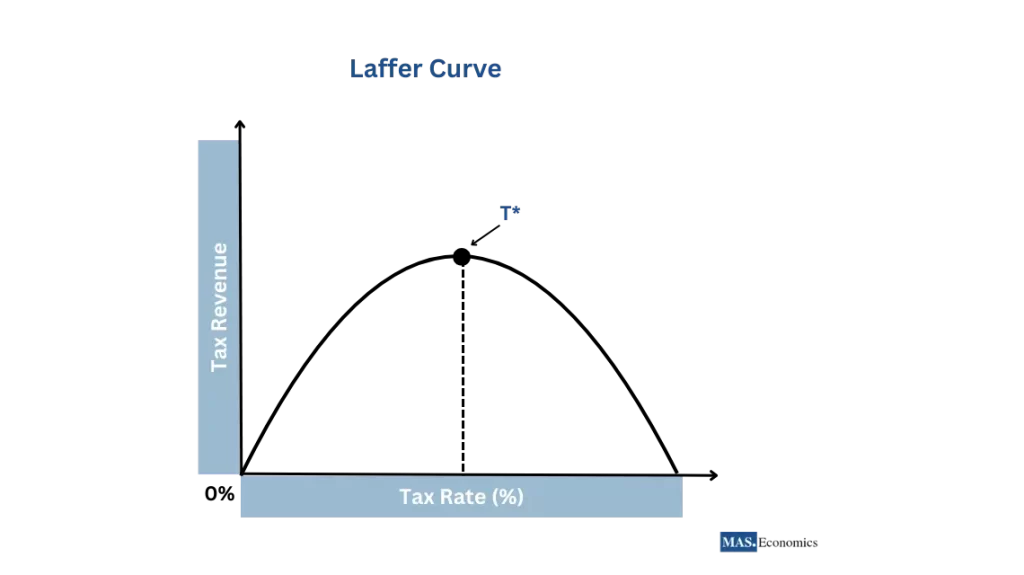
If you look at the Laffer curve above, you can see that as the tax rate increases from 0%, the government’s revenue also increases. The upward slope of the curve indicates this. Revenues continue to increase until they reach a point called T*, which is the top of the curve. This point represents the optimal tax rate. As can be seen from the downward slope of the curve beyond T*, increasing tax rates reduces revenue. This decline is due to the negative effects of higher tax rates on economic behavior, such as reduced work effort, lower investment, tax evasion, and other distortions that can lead to a smaller tax base.
The Laffer Curve has been influential in shaping the tax policy debate. Policymakers often try to find the sweet spot on the curve where tax rates are high enough to generate significant revenue, but not so high that they discourage economic activity. However, the exact shape of the Laffer curve and the optimal tax rate remain subject to debate, as they may vary depending on the specific tax, time period, and economic circumstances.
J curve
The J-curve concept emerged in the 1970s as economists observed the lagged effect that currency depreciation had on a country’s trade balance. The shape of the graphical representation of this relationship gave rise to the term “J curve.”
The J curve describes the trajectory of a country’s trade balance as its currency depreciates. Initially, imports become more expensive in domestic currency, while exports remain unchanged in foreign currency, worsening the trade balance. Over time, as exports become more competitive and import demand declines, the trade balance improves, forming a characteristic J-shaped curve.

The J curve above illustrates how a country’s trade balance (vertical axis) changes after a currency weakens (horizontal axis). The J shape reflects the initial and final effects of depreciation.
Initially, the trade balance deteriorates (J-shaped downward part). Imports become more expensive for domestic consumers, while exports remain the same for foreign buyers. Over time (right side of J), this often leads to a trade deficit because import costs outweigh export revenues and circumstances change. Cheaper exports become more attractive to foreign buyers, potentially increasing demand. Additionally, domestic consumers may reduce their purchases of expensive imported goods. These factors can lead to trade surpluses where the value of exports exceeds the value of imports.
The speed and extent of improvement depends on a variety of factors. factorand how sensitive demand is to price changes (elasticity) and overall economic conditions.
The J-curve effect has been observed in a variety of historical and contemporary cases. For example, after the Plaza Agreement in 1985, the U.S. trade balance initially worsened and then improved as the U.S. dollar depreciated against the Japanese yen. Recently, the decline in the value of the British pound following the 2016 Brexit referendum showed a similar pattern, with the trade balance initially deteriorating and then gradually improving.
Kuznets curve
The Kuznets curve, named after American economist Simon Kuznets, was introduced in the 1950s. Kuznets hypothesized that as economies develop and industrialize, income inequality follows an inverted U-shaped pattern.
The Kuznets curve suggests that income inequality increases in the early stages of economic development as the economy transitions from a rural agricultural society to an urban industrial society. As development continues and the benefits of growth spread more broadly, income inequality declines, forming an inverted U shape.

The Kuznets curve described above depicts the relationship between income inequality (vertical axis) and national economic development measured by GDP per capita (horizontal axis). The curve follows an inverted U shape.
Less developed economies exhibit lower income inequality at the starting point (Y1). As the pace of development accelerates (Y2), the gap between rich and poor widens. This is due to the increased specialization and concentration of wealth among those who own capital (land, factories). However, the curve begins to curve downward after reaching the turning point (Y2). As development continues, government policies such as redistribution programs, investments in education and training, and technological advancements can help spread the benefits of growth more broadly, ultimately reducing income inequality (Y3).
The Kuznets curve has been a subject of debate among economists. Some argue that the relationship between economic development and inequality is more complex and varies across countries and periods. Additionally, the relevance of the curve in modern post-industrial economies has been questioned because factors such as globalization, technological change, and public policy can have a significant impact on income distribution.
Lorenz curve
Developed by American economist Max Lorenz in the early 20th century, the Lorenz curve is a graphical representation of income inequality within a population. It has become a fundamental tool for studying income and wealth distribution.
The Lorenz curve shows the cumulative proportion of total income received by cumulative proportions of the population, from the poorest to the richest. The diagonal line represents perfect equality, with each population receiving the same proportion of income. The greater the distance between the Lorenz curve and the diagonal, the higher the income inequality.

The equality line (45 degrees) is the diagonal line that represents perfect income equality. If everyone earned exactly the same amount, the income distribution would follow this line. That is, for example, the bottom 20% of the population would earn 20% of the total income, and the bottom 50% would earn 50%. to. The bow-shaped line represents the actual income distribution. It plots the cumulative ratio of total income (vertical axis) to the cumulative proportion of the population (horizontal axis), starting with the poorest individuals or households.
The area A between the equality line and the Lorenz curve represents the degree of income inequality. The more important area A is, the larger the gap becomes. The Gini coefficient, another measure of income distribution, can be calculated as the ratio of the area of A to the sum of the areas of A and B in the Lorenz curve.
conclusion
These key macroeconomic curves (Phillips, Laffer, J, Kuznets, and Lorenz) provide a springboard for analyzing key economic relationships. Simplifies complex concepts like inflation, unemployment, and income inequality.
Remember that this is a simplified model. The real world is more nuanced with factors affecting the shape and stability of these curves. Consider the Phillips curve. This highlights short-term trade-offs, but longer-term dynamics and inflation expectations may change this.
Similarly, the Laffer Curve suggests the optimal tax rate, but economic conditions and tax structures make it difficult to pinpoint. The J curve and Kuznets curve provide insight into trade and development, but globalization and policies can influence these relationships.
The Lorenz curve is a visual representation of income inequality. However, the Gini coefficient provides a single measure, and a deeper understanding requires examining the root causes of inequality.
Thanks for reading! If you found this insightful, share the knowledge with your friends and spread the word on social media!
Happy learning with MASEconomics






